
 |
 |
| Addresses 2 and 4 appear to have about the same number of points. Addresses 1 and 3 have about the same number of points, and fewer than in addresses 2 and 4. So we think | |
| p2 = p4 > p1 = p3 | |
| Because r1 = r2 = r3 = r4, the minimum probability corresponds to the maximum α, and the maximum probability corresponds to the minimum α. | |
| Then | |
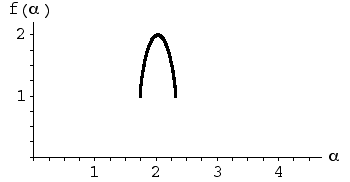
| The maximum probability occurs when just the transformations T2 and T4 are applied. These generate the line between corners 2 and 4, so this line fills in most densely, which we observe. Then f(αmin) is the dimension of the line between corners 2 and 4, that is, f(αmin) = 1. We see this on the left endpoint of the f(α) curve. | |
| The minimum probability occurs when just the transformations T1 and T3 are applied. These generate the line between corners 1 and 3, so this line fills in least densely, which we observe. Then f(αmax) is the dimension of the line between corners 1 and 3, that is, f(αmax) = 1. We see this on the right endpoint of the f(α) curve. | |
| The four transformations T1, T2, T3, and T4 generate the filled-in unit square, a 2-dimensional shape. consequently max(f(α)) = 2. We see this as the highest point of the f(α) curve. | |
| Here we see less diffrence in density between squares 1 and 2 than we saw in the previous example, so here p1 and p2 are closer than they are in the previous example, and consequently αmin and αmax are closer together here than in the previous example. | |
Return to visual interpretations of f(α) curves.